Content
Simple
Planar Truss
Determination of Forces in Trusses :
Solving Equilibrium Equations:
Solving Static Equilibrium:
Solving Force Vector Diagram:
Simple Planar Truss
Determination of Forces in Trusses :

Eight equations with eight unknowns imply the forces in the truss is statically determinate. There are a number of way to determine forces in trusses by the method of joints.
Solving Equilibrium Equations:
In general the eight equilibrium equations can be solved mathematically. The eight equilibrium equations can be divided into two groups, i.e. with external reactions and without external reaction. Imply
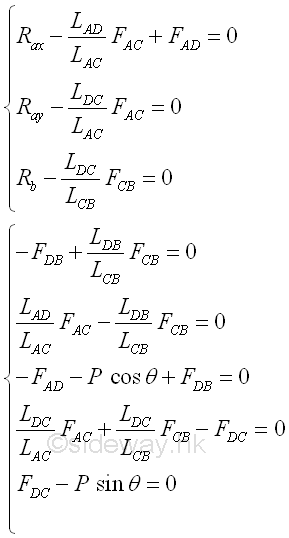
Focusing on the group without external reaction, five equations with five unknowns imply the forces in the truss member can be determinated.
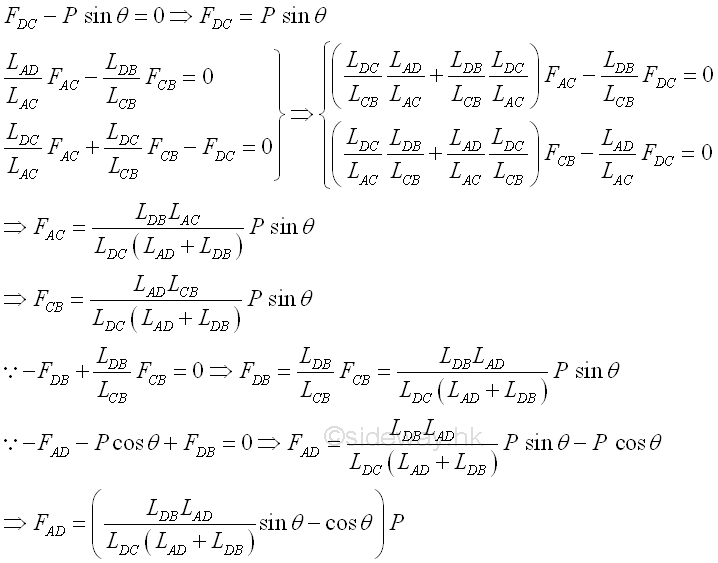
The external reaction can then be determined. Imply
Solving Static Equilibrium:
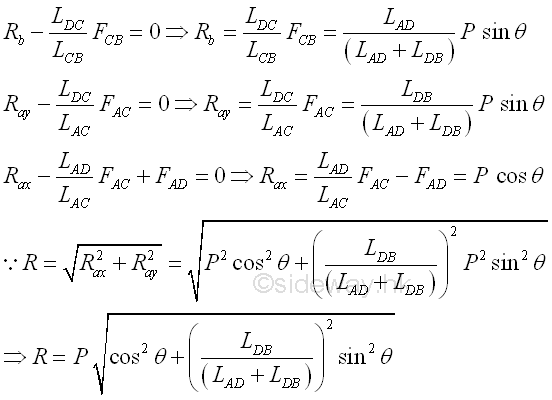
Or unknown forces can also be determined by making use of the mechanical static equilibrium state. When the completely constrained truss is in static equilibrium, all the unknown reactions can be determined. Imply
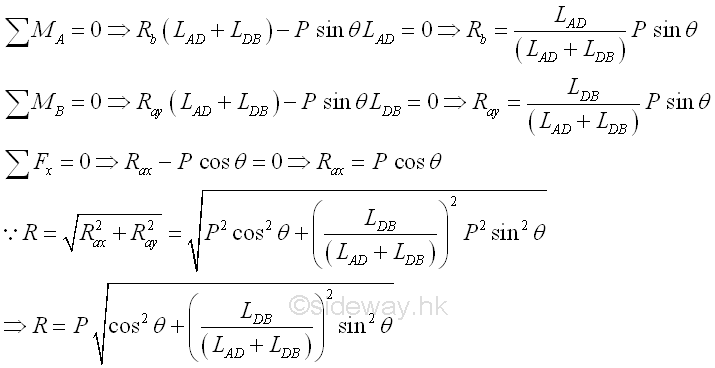
Since a simple truss is constructed by adding two truss members to form a new joint, in general, a joint involving two unknown forces from two truss members can always be found. For a statically determinate truss, the unknown forces in the truss can always be determined by the static equilibrium of the joint pin connecting to two truss members. Therefore starting with a joint with two truss members, i.e. joint pin A. Imply
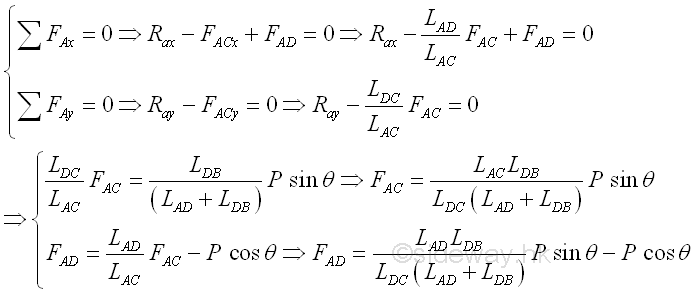
After two unknowns are determined, the unknowns of the two neighborhood joints will be reduced by one. Applying the same strategy to select neighborhood joints with two unknowns, i.e. joint pin D. Imply
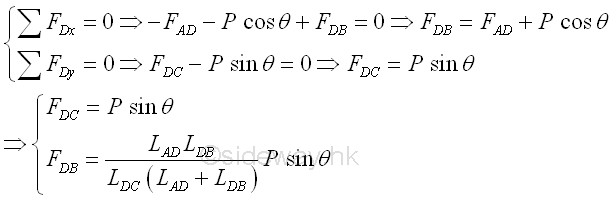
Repeating the procedure untill all unknown are determined. All possible joints with two unknowns i.e. joint pins B and C can be used. It can be either to continue selecting the neighborhood joint or to start with other possible joint from other ends. Use joint C, Imply

Solving Force Vector Diagram:
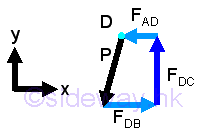
Sometimes, the unkonwn forces can be determined using graphical methods through the determination of force vector by constructing the force vector diagram at each joint. Starting the joint pin with known force, so that all force vector diagram can be scaled to a known value, i.e joint pin D, Although joint pin D is constained in y dimension only, joint pin D is still a good choice. Besides the equilibrium force vector diagram should always be draw in one direction for all joint pins, i.e. counter-clockwise direction imply
Continuing the joint pin with known dimension, which is fully constrained by the known force before so that all force vector diagram can be scaled to a known value, i.e joint pin C, imply
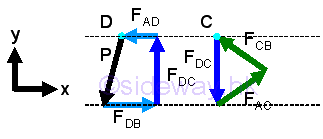
Continuing the joint pin with known dimension, which is fully constrained by the known force before so that all force vector diagram can be scaled to a known value, i.e joint pin B. The vector diagram of Joint pin A is not fully constrained because the unknown reaction R is a vector of two rectangular components. imply
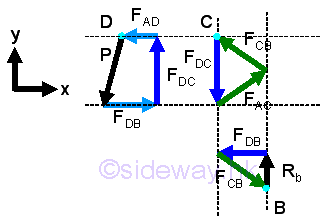
Since FDB is constrained, the unconstrained force vectors at point D can be fixed also. imply
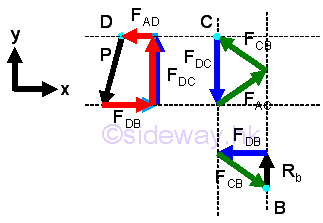
Continuing the joint pin with known dimension, which is fully constrained by the known force before so that all force vector diagram can be scaled to a known value, i.e joint pin A. imply
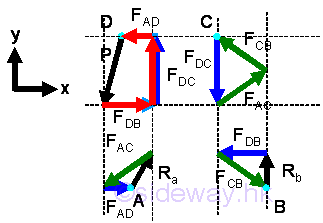
The force vector diagtram can be joined to form a single diagram, known as Maxwell's diagram. This single diagram can give a more general idea on the characteristic of the trusses, imply
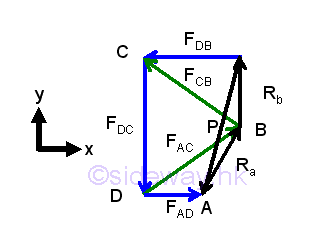
©sideway
ID: 120200072 Last Updated: 2/26/2012 Revision: 0 Ref:
References
- I.C. Jong; B.G. rogers, 1991, Engineering Mechanics: Statics and Dynamics
- F.P. Beer; E.R. Johnston,Jr.; E.R. Eisenberg, 2004, Vector Mechanics for Engineers: Statics
Latest Updated Links
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)
- Itinerary Okinawa 13 day(last updated On 1/15/2025)
- Itinerary Okinawa Bus Yanbaru Express Bus(last updated On 1/10/2025)
- Itinerary Okinawa Bus DAIICHI KOUTSU(last updated On 1/9/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 254
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
