Content
Summation of Accumulative Physical Quantity
Work by a Force
Work to
compress or stretch a spring
Work to move an object
Work to lift a load
with a light wire
Work to lift a
variable load
Work to lift a load with a heavy cable
Work to raise fluid
Work to
raise fluid in a cylindrical tank
Work to
raise fluid in a conical tank
Summation of Accumulative Physical Quantity
Similar to the extension of finding accumulative physical quantity from area to volume, the application of integration can be used to calculate some variable mechanical quantity by expressing the mechanical quantity in the form of accumulative mechanical quantity.
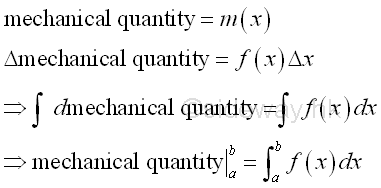
Work by a Force
Work can be defined as the product of a force acting upon an object and the displacement of the object caused by the applied force.
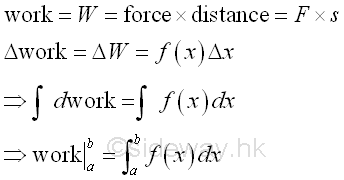
For a constant force F, imply

Work to compress or stretch a spring
For a variable force f(x) such as spring force, the force varies with the length of spring,
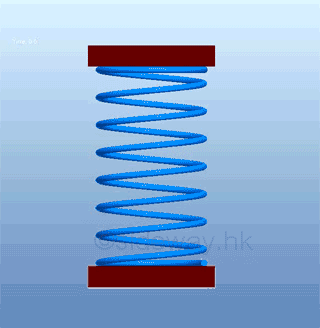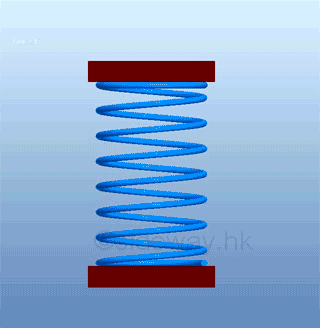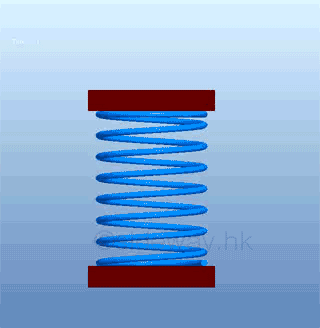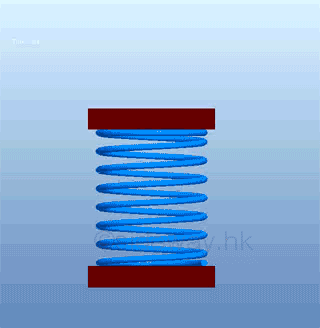
The force of a spring is proportional to the length of compression or extension from its natural length. Graphically, the work done by spring is,

For a spring of natural length 20 with k equals to 1, the force when the length of spring is compressed to 16 is equal to 4. The distance and work done of spring compresssion is therefore equal to

And geometrically, the work is equal to the area under the curve, that is half of the product of force and travelled distanct, (kx*x)/2.
For a spring of natural length 20 with k equals to 1, the distance and work done of spring compression from 16 to 12 is equal to
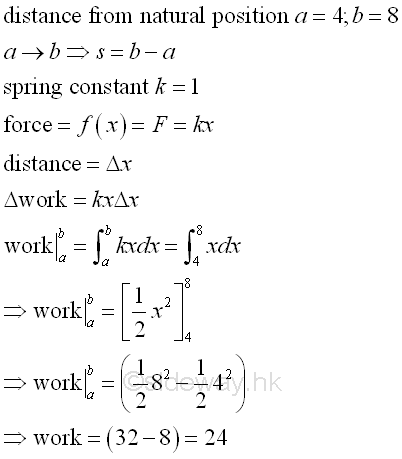
Both the applied force and the displacement always have the same direction in compressing or stretching the spring , they have the same sign. Therefore, for a spring of natural length 20 with k equals to 1, the distance and work done of spring extension from 24 to 28 is same as the work done of spring compression from 16 to 12.
Work to move an object
Work done on an object is equal to the travelled distance multiplied by the applied force along the travelling direction. In general, the amount of work done by an applied force to move an object with equal displacement along the applied force in any direction is the same.
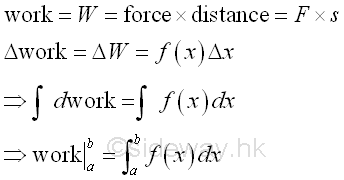
Work to lift a load with a light wire
For a light wire, the weight of the wire can be neglected. The total work done is obtained by slicing the work into infinitesimal work elements. Since the applied force to lift the load is a constant, the amount of work done is equal to the applied force times the displacement.

Work to lift a load of 20 from ground 0 to a 30 high platform is.
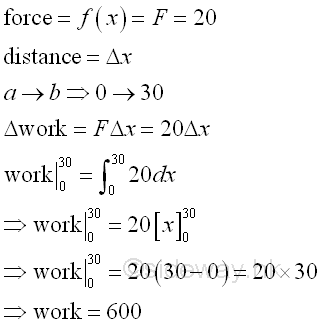
Work to lift a variable load
For a light wire, the weight of the wire can be neglected. If the load is a leaky sandbag with leaking rate 0.1 per unit travelled length, the load becomes a variable load. The applied force to lift the load decreases with the height because of sand leaking. The total work done is obtained by slicing the work into infinitesimal work elements. The amount of work done is equal to the summation of the infinitesimal work elements.
Work to lift a leaky load of 20 from ground 0 to a 30 high platform is.

Work to lift a load with a heavy cable
For a heavy cable with 0.1 per unit length, the weight of the cable can not be neglected. The applied force to lift the load should include the weight of the lifting cable, therefore the total load is decreasing while lifting the object because the length of cable is reducing. Unlike the concentrated load, the cable load is a distributed load along the lifting direction, therefore the travelled distance for each infinitesimal load element is not the same and all infinitesimal work elements by each infinitesimal load element should be calculate individually. And the total work done is then obtained by the summation of all infinitesimal work elements by each infinitesimal load element and the concentrated load.
Work to lift a load of 20 from ground to a 30 high platform is.
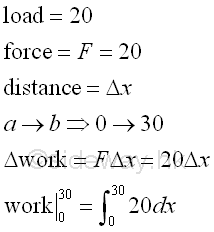
Work to lift a heavy cable with 0.1 per unit length from ground to a 30 high platform is.
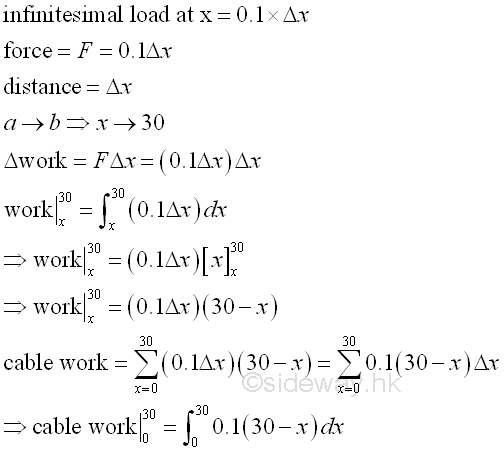
Total work to lift a load of 20 with a heavy cable with 0.1 per unit length from ground to a 30 high platform is
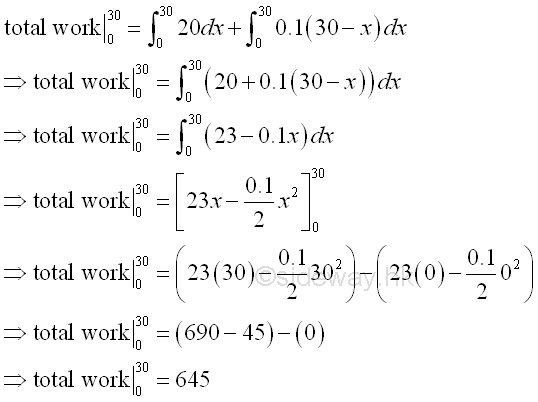
Work to raise fluid
Similar to lifting a heavy cable, raising fluid in a tank can be considered as moving fluid layer by layer, therefore problem of raising fluid from a tank to a higher level is also a problem of moving a distributed load.
Work to raise fluid in a cylindrical tank
For a cylindrical tank, the cross-sectional area of the tank is equal, therefore the work done to raise fluid for every infinitesimal layer element is the same.
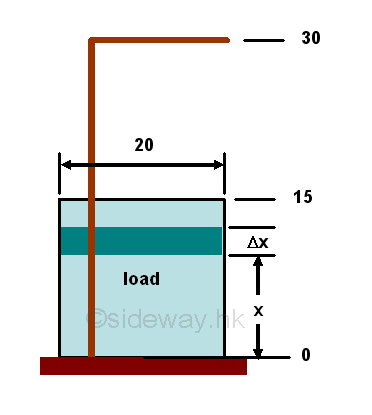
Work to raise a layer of density 1 per unit volume fluid from a cylindrical tank of diameter 20 with fluid height 15 to a level15 above the tank, that is a 30 high from the ground is.
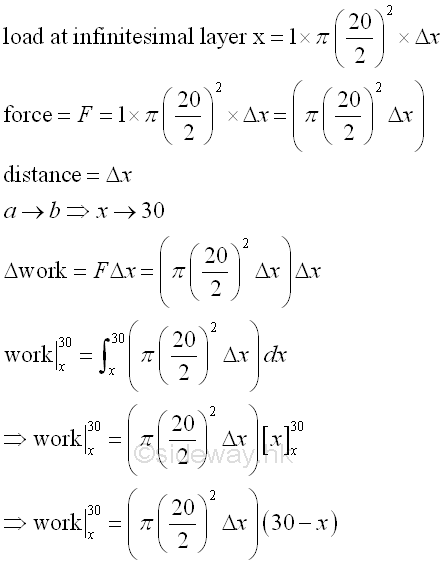
Total work to raise all fluid from 0 to15 in the tank to a level 15 above the tank is.

Work to raise fluid in a conical tank
For a inverted conicall tank, the cross-sectional area of the tank varies with height, therefore the work done to raise fluid for every infinitesimal layer element varies with height also
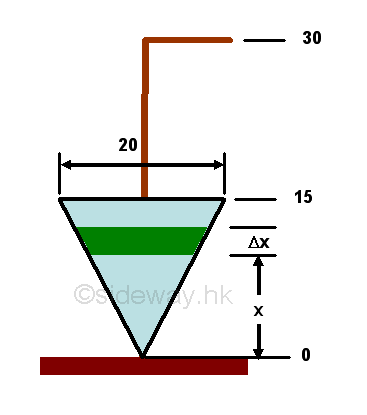
Work to raise a layer of density 1 per unit volume fluid from a conical tank of base diameter 20 with fluid height 15 to a level15 above the tank, that is a 30 high from the ground is.
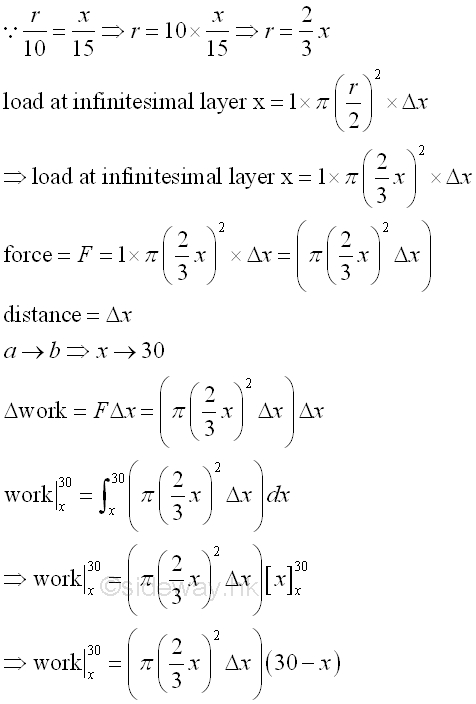
Total work to raise all fluid from 0 to15 in the tank to a level 15 above the tank is.

©sideway
ID: 111200004 Last Updated: 12/13/2011 Revision: 0 Ref:
References
- S. James, 1999, Calculus
- B. Joseph, 1978, University Mathematics: A Textbook for Students of Science & Engineering
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
